Latest revision 07-02-2024
index
$
\def \hieruit {\quad \Longrightarrow \quad}
\def \met {\quad \mbox{with} \quad}
\def \EN {\quad \mbox{and} \quad}
\def \SP {\quad ; \quad}
\def \half {\frac{1}{2}}
$
Let's do it this way, as I found out last night.
Symbols: $t=$ measured (atomic) time, $H_0=$ Hubble parameter, $z=$ cosmological redshift.
$$
t = \frac{1}{H_0}\ln\left(\frac{1}{1+z}\right) \quad \Longrightarrow \quad dt = -\frac{1}{H_0}\frac{dz}{1+z}
$$
And $\tau=$ Minkowsi time.
$$
H_0\tau+1 = \frac{1}{1+z} \quad \Longrightarrow \quad d\tau = -\frac{1}{H_0}\frac{dz}{(1+z)^2}
$$
Combining the two gives
$$
dt = (1+z).d\tau
$$
In this way the Measured time tick is indeed a time dilation of the Minkowski time tick with the Time Dilated Past hypothesis.
The Variable Mass Theory gives a slightly different outcome, namely
$$
\frac{dT}{dt} = \sqrt{\frac{m}{m_0}} \quad \mbox{with} \quad 1+z = \frac{\lambda}{\lambda_0} = \frac{m_0}{m}
$$
And so
$$
dt = \sqrt{1+z}.dT
$$
Fortunately, we use the same symbol for Measured Time = Atomic Time. But Minkowski Time $\tau$ is not the same as Orbital Time $T$. Or is it?
Nevertheless we both arrive at similar formulas by joining the two time scales. Consistency check:
$$
t=\frac{1}{H_0}\ln\left(\frac{1}{1+z}\right)=\frac{1}{H_0/2}\ln\left(\sqrt{\frac{1}{1+z}}\right)=\frac{1}{H_0/2}\ln\left(H_0/2.T+1\right)
$$
$$
H_0/2.T+1=(1+z)^{-1/2} \quad\Longrightarrow\quad dT=\frac{1}{H_0/2}(-1/2)\frac{dz}{(1+z)^{3/2}}
$$
$$
dt=-\frac{1}{H_0}\frac{dz}{1+z} \quad\wedge\quad dT=-\frac{1}{H_0}\frac{dz}{(1+z)\sqrt{1+z}} \quad\Longrightarrow\quad dt=\sqrt{1+z}.dT
$$
For values much smaller than the Hubble time the different time spans cannot be distinguished from each other:
$$
\left(\frac{1}{2}H_0 T+1\right)^2=H_0\tau+1=e^{H_0 t}
$$
$$
1+H_0 T \approx 1+H_0 \tau \approx 1+H_0 t
$$
And all time scales share the same value for the Hubble parameter.
@mikehelland
There exists a critical essay, written by Léon Brillouin at the end of his life. The title of the booklet is Relativity Reexamined. Quoting from page 33: Einstein's clocks were supposed to emit extremely short signals and to accurately measure time intervals between signals emitted and received. In a word, an Einstein clock was a radar system, and its requirements were thus very different from those of a frequency standard. Your "light clock" is such a radar system. But okay, that's not necessarily bad.
Question here is consistency. Let's see, departing from the formulas we do agree upon.
$$
-t = \frac{1}{H}\log(1+z) \quad ; \quad \tau = \frac{1}{H}\left(e^{Ht}-1\right)
$$
And so
$$
\tau = \frac{1}{H}\left(e^{H.-1/H\log(1+z)}-1\right) = \frac{1}{H}\left(\frac{1}{1+z}-1\right) = -\frac{1}{H}\frac{z}{1+z}
$$
Hence - inevitably - the associated (Minkowski) distance is, indeed
$$
d = -c\tau = \frac{c}{H}\frac{z}{1+z} \quad \mbox{and} \quad 0 \le d \le \frac{c}{H}
$$
There is an independent Cosmic distance ladder though. Are you sure that these distances are limited to the Hubble length $c/H \approx$ 14.4 billion light-years ?
Update
For the (HdB version of) the Variable Mass Theory we find analogously the following.
$$
-t = \frac{1}{H}\ln(1+z) \quad ; \quad \left(1+\frac{1}{2}H.T\right)^2 = e^{H.t}
$$
And so
$$
1+\frac{1}{2}H.T = e^{H/2.t} = e^{H/2.-1/H.\ln(1+z)} = e^{\ln(1/\sqrt{1+z})} = \frac{1}{\sqrt{1+z}}
$$
Hence for the accompanying "Proper distance":
$$
d_P = -c.T = \frac{2c}{H}\left(1-\frac{1}{\sqrt{1+z}}\right) \quad \mbox{and} \quad 0 \le d_P \le \frac{2c}{H}
$$
Oh my, that is an interesting "coincidence" !! Let's take a look again at slide 47 as displayed in thread 188.
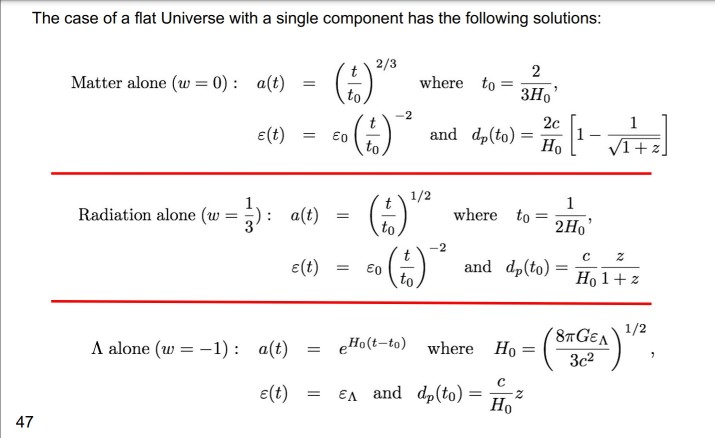
Then we see that
- The (HdB version of) the Variable Mass Theory corresponds with a BB flat Universe with Matter alone
- The Time Dilated Past hypothesis by @mikehelland corresponds with a BB flat Universe with Radiation alone
- Eric Lerner's Static Euclidean Universe corresponds with a BB flat Universe with Λ alone (and $d_P=c/H.z$ )
@ExpEarth writes:
Suppose that the speed of light steadily diminishes with distance.
With the variable mass theory it is exactly the other way around. The speed of light steadily increases with distance, in some sense.
Mathematically. With $t=$ atomic time, $T=$ orbital time and $H=$ (intrinsic) Hubble parameter we have
$$
\frac{1}{2}H.t = \ln\left(1+\frac{1}{2}H.T\right) \quad \Longrightarrow \quad \frac{dt}{dT} = \frac{1}{1+H/2.T}
$$
On the other hand, with $m=$ variable mass and $_0=$ here and now, according to Narlikar's law:
$$
\frac{m}{m_0} = \left(1+\frac{1}{2}H.T\right)^2 \quad \Longrightarrow \quad \frac{dt}{dT} = \sqrt{\frac{m_0}{m}} = \sqrt{1+z}
$$
The speed of light $c$ (orbital) or $c_0$ (atomic) can be expressed with the two time scales. Let $s=$ distance, then
$$
c_0 = \frac{ds}{dt} \quad \mbox{and} \quad c = \frac{ds}{dT} = \frac{ds}{dt}\frac{dt}{dT} = c_0\sqrt{\frac{m_0}{m}} = c
$$
According to the variable mass theory, mass is increasing with time.
Therefore $c$ in the past has been larger than it is now, because of $m_0 \gt m$ , in some sense (: orbital frame).
It follows that (mass)energy, as calculated within the orbital time frame, is conserved.
$$
\large \boxed{mc^2=m_0c_0^2}
$$
(supposing we are near the universe centre).
You are saying this repeatedly. But, Matt, we are always in the centre of our own observable part of the universe.
So we could have redshift linked to time dilation and mass variation.
Yes, atomic clocks with lighter electrons run slower and send redshifted light.
Note.
From Relativity Reexamined by Léon Brillouin:
We witnessed the invention of atomic clocks of incredible accuracy, whose physical properties differ very much from the clocks Einstein imagined [ i.e. the "light clocks" as employed by @mikehelland ]. Let us mention here a real difficulty resulting from internationally adopted definitions. The unit of length is based on the wavelength of a spectral line of krypton-86 under carefully specified conditions with accuracy $10^8$ and the unit of time is based on the frequency of a spectral line of cesium with accuracy $10^{12}$. Hence, the same physical phenomenon, a spectral line, is used for two different definitions: length and time, and the velocity $c$ of light remains undefined and looks arbitrary. It should be stated, once and for all, whether a spectral line should be used to define a frequency or a wavelength, but not both!
Meanwhile, the evil has been done ! Spectral lines are used for both length and time. And thus defined these are associated with atomic time, not with orbital time. As a consequence, the lightspeed $c_0$ in the atomic timeframe is a constant by definition, not only here and now, but a universal constant. We have done it ourselves; it's not a "law of nature".
@budrap00 writes:
the FLRW mistake of assuming a "universal metric" with physically meaningful space and time coordinates.
Exactly: metric is not physic !!
@mikehelland :
I do not understand why you are stoking another little campfire that seems to replace your Time Dilated Past hypothesis.
In a Static Euclidean infinite and eternal Universe there are many timescales that show a Time Dilated Past:
$$
t=\frac{1}{H}\ln\left(\frac{1}{1+z}\right)=\frac{p}{H}\ln\left(\left[\frac{1}{1+z}\right]^{1/p}\right)=\frac{p}{H}\ln\left(H/p.\tau+1\right)
$$
$$
H/p.\tau+1=(1+z)^{-1/p} \quad\Longrightarrow\quad d\tau=\frac{p}{H}(-1/p)\frac{dz}{(1+z)^{1+1/p}}
$$
$$
dt=-\frac{1}{H}\frac{dz}{1+z} \quad\wedge\quad d\tau=-\frac{1}{H}\frac{dz}{(1+z)(1+z)^{1/p}} \quad\Longrightarrow\quad \large \boxed{dt=(1+z)^{1/p}.d\tau}
$$
The special cases $p=1$ and $p=2$ have been covered in that previous post.
@RedshiftDrift .
What's interesting is which metric you choose as being the one with physical reality.
Very much agreed !! This is more or less similar to my findings in a previous posting.
To my surprise, there are infinitely many time scales that lead to the well-known distance formula $r=c/H\ln(1+z)$.
Therefore it's impossible to decide - based upon that formula - which Time Dilated Past would be the physical relevant one. Mike's favorite is $dt=d\tau(1+z)$ ; my favorite is $dt=dT\sqrt{1+z}$ ; other favorites are thinkable.
Update
A nice example of a wrong reference frame in everyday physics is the following problem: Strapping down a cylinder.
The author of the accepted answer says that the length of the string is
$$
l(\alpha)=2\sqrt{h^2+r^2\alpha^2}+2r\cos\alpha
$$
with $r=$ radius, $h=$ height, $\alpha=$ angle. Crucial is the remark that $r\alpha$ comes from coordinates on the unfolded cylinder.
But the cylinder is not unfolded in todays physical reality.
As my comment says: Almost, but not quite. Pythagoras is not for triangles with an arc in it. We must have instead
$$
l(\alpha)=2\sqrt{h^2+4r^2\sin^2(\alpha/2)}+2r\cos\alpha
$$
For small angles $\alpha$ the lengths $l$ are indistinguishable.
Let $H=$ Hubble parameter, $G=$ gravitational constant, $\rho_0=$ mass density here and now, OI = from Origin of Inertia, the book by Amitabha Ghosh, Mach = another value from the same book, VMT = version of the Variable Mass Theory (and ΛCMD = oh well). Then we have
$$
H = \sqrt{\chi G \rho_0} \quad \mbox{with} \quad \chi = 8\pi \times
\begin{cases}
1/8 & (: \mbox{OI}) \\
1/6 & (: \mbox{Mach}) \\
1/3 & (: \Lambda\mbox{CDM}) \\
0.3883945571 & (: \mbox{VMT})
\end{cases}
$$
But there are other theories about the Hubble parameter saying that
$$
H =
\begin{cases}
2/\mbox{age} & (: \mbox{VMT}) \\
1/\mbox{age} & (: \mbox{other})
\end{cases}
$$
Let us assume that the first set of formulas is correct.
According to the Variable Mass Theory, mass is increasing with time. Consequently, in a Static Euclidean Universe, the mass density must be increasing with time as well. And so the Hubble parameter must be increasing with time.
However, according to the second set of formulas, the Hubble parameter is inversely proportional with time and so, because time is always increasing, the Hubble parameter must be decreasing with time.
Contradiction. What is it?
Indeed. According to (not only) ΛCDM theory we have, with $R=$ radius (of comoving volume), $A=$ (universe) age, $c=$ lightspeed, $H=$ Hubble parameter:
$$
R = \frac{c}{H} \quad \mbox{and} \quad A = \frac{1}{H}
$$
And that would be valid only for that special moment when time from present is precisely zero, therefore only here and now? It makes ΛCDM an unbelievable theory, literally.
Looking for feedback on metric for the universe, with time dilation but no expansion has made possible other interesting discoveries.
The formulas in the post as linked can be written as follows, with $t=$ measured time (negative), $\tau=$ Minkowski time, $H=$ Hubble parameter and $p\rightarrow n$ (what's in a name).
$$
H/p.\tau+1=(1+z)^{-1/p}=\left[e^{-Ht}\right]^{-1/p} \quad \mbox{or} \quad 1+H/n.\tau = e^{H/n.t}
$$
One consequence is that time $\tau$ is an approximation for time $t$ which becomes better for larger values of $n$ (why didn't I see before now this beautiful relationship with pure mathematics).
$$
\left(1+\frac{H\tau}{n}\right)^n = e^{H.t} \quad \mbox{with} \quad \lim_{n\to\infty} \left(1+\frac{x}{n}\right)^n = e^x
$$
Another consequence is that there must be a beginning / a moment of creation A(lpha) in the Minkowsy time frame for $\tau = -n/H = -A$ , but not in the measured time frame $t$ . The end-result becomes extremely simple.
$$
1 + \tau/A = e^{t/A}
$$
This means that Orbital time $T$ as defined in (my version of) the Variable Mass Theory is exactly the same as Minkowski time $\tau$ as defined in Mike Helland's theory, provided that the Hubble parameter is based on age only according to the above. Many results in my theory can thus be transferred to Mike's theory and vice versa. Very nice !! To be continued ..
Let us resume with the formula we ended with; let $A=$ age, $t=$ atomic time, $\tau=$ orbital time.
$$
1+\tau/A = e^{t/A} \quad \Longleftrightarrow \quad t = A\ln(1+\tau/A)
$$
Now calculate, for example, velocities $v$ and accelerations $a$ from displacements $s$ within the two time frames.
$$
\vec{v}_\tau = \frac{d\vec{s}}{d\tau} = \frac{d\vec{s}}{dt}\frac{dt}{d\tau} = \vec{v}_t\frac{1}{1+\tau/A}
$$
$$
(1+\tau/A)\vec{v}_\tau = \vec{v}_t
$$
Patiently differentiating further with product rule and chain rule gives
$$
\vec{a}_\tau = \frac{d^2\vec{s}}{d\tau^2} = \frac{d}{d\tau}\left(\frac{d\vec{s}}{dt}\frac{dt}{d\tau}\right) = \frac{d}{d\tau}\left(\frac{d\vec{s}}{dt}\right)\frac{dt}{d\tau} + \frac{d\vec{s}}{dt}\frac{d}{d\tau}\left(\frac{dt}{d\tau}\right) = \frac{d^2\vec{s}}{dt^2}\left(\frac{dt}{d\tau}\right)^2 + \frac{d\vec{s}}{dt}\frac{d^2t}{d\tau^2}
$$
Hence
$$
\vec{a}_\tau = \vec{a}_t\left(\frac{1}{1+\tau/A}\right)^2 + \vec{v}_t\frac{-1/A}{(1+\tau/A)^2}
$$
$$
(1+\tau/A)^2 \vec{a}_\tau = \vec{a}_t - \vec{v}_t/A
$$
Under eartly circumstances here and now we have that $\tau=0$ and so
$$
\vec{v}_\tau = \vec{v}_t
$$
$$
\vec{a}_\tau = \vec{a}_t - \vec{v}_t/A
$$
Conclusion: velocities in the two time frames are the same, but accelerations are not.
An application
A quote from the book Origin of Inertia by by Amitabha Ghosh: It is now an established fact that the spin motion of the Earth is gradually slowing down, and the magnitude of this secular retardation is about $6 \times 10^{-22}\mbox{ } rad/s^2$ . That amount of information is already sufficient. According to our theory we have - as repeated from the above:
$$
\vec{a}_\tau = \vec{a}_t - \vec{v}_t/A
$$
Here $\vec{a}_\tau$ is the "true" Newtonian acceleration (namely in orbital time), $\vec{a}_t$ is the acceleration as measured nowadays with atomic clocks and $A$ is an age. All accelerations are proportional with $R=$ radius of the earth. If there is a discrepancy $\Delta\omega$ between the orbital and atomic angular velocities, then it must being caused by the negative term, which is a vector in tangential direction slowing down the rotation. The calculation is a one-liner.
$$
-|\vec{v}_t |/A = -\omega R/A \quad \Longrightarrow \quad \dot{\omega} = -\left(\frac{2\pi}{\mbox{day}}\right)/\mbox{age}
$$
# Age of the earth
age := 4.543*10^9;
# Seconds in a year
year := 31556926;
# Seconds in a day
day := 60*60*24;
# Rotation of the earth
omega := 2*Pi/day;
# Retardation
slower := evalf(omega/(age*year));
We find that the retardation is about $5 \times 10^{-22} \mbox{ } rad/s^2$
@mikehelland .
Just one small detail:
$$
R = \frac{c}{H} * 3.261564
$$
A slightly different value (with underpinning) is presented in the G. de Vaucouleurs section at my website:
$$
R = \frac{c}{H} * 3.383634283
$$
Also take notice of the fact that I'm no longer saying that $H = 1/A$ or $H = 2/A$ but instead that the Hubble parameter is inversely proportional to Age, with an arbitrary factor $n$, not even necessarily an integer: $H = n/A$ .
