by Frank H. Shu
In my humble opinion - I'm not an astronomer - it's a bit suspect that anything in the distant
cosmos appears to be oversized. And the more distant, the more oversized. It's not impossible that
flaws in the Cosmic Distance Ladder (Wikipedia) are to be hold responsible for this, as is argued in Incorrect Assumptions in Astrophysics. While this might provide an explanation for
the issues (1), (3) and (4) in Box 12.1, it definitely does not clarify issue (2): the flat
Galaxy rotation curves:
At an alternative website, called The 100 Year Wrong Turn in Cosmology, we find a PDF document with the intriguing title "Galactic mass distribution without dark matter or modified Newtonian mechanics". It is written by Kenneth F Nicholson, a retired engineer. A more elaborate paper with analogous content is the PDF titled "Mass Distribution in Rotating Thin-Disk Galaxies According to Newtonian Dynamics", by James Q. Feng and C. F. Gallo. A very much improved version of the latter paper is:
According to Newton's law of universal gravitation, the attractive force ($F$) between two bodies is directly proportional to the product of their masses ($m_1$ and $m_2$), and inversely proportional to the square of the distance ($r$ : inverse-square law) between them (see Wikipedia): $$ F = G\frac{m_1 m_2}{r^2} $$ The constant of proportionality ($G$) is the gravitational constant: $$ G = 6.674\,08(31) \times 10^{-11}\,m^3\,kg^{-1}\,s^{-2} $$ Newton's law of gravitation shall be applied to a RingWorld of gravitating matter, to begin with.
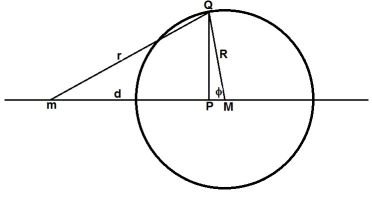
Let $m$ be a test mass at some distance $d$ from the center $M$ of the RingWorld.
Let $Q$ be the position of an infinitesimal element in the Ring.
The mass $m_1$ in Newton's law shall be identified with the test mass $m$.
Let the mass density (with dimension $kg/m^2$) in the (flat) ring be equal to $\rho$.
Then the infinitesimal element at $Q$ has a mass $\rho\,R\,dR\,d\phi$ , to be identified with $m_2$.
So Newton's law becomes, for the gravitational attraction between the test mass and an infinitesimal
part of the ring:
$$
dF = G\frac{m \times \rho\,R\,dR\,d\phi}{r^2}
$$
Where $r$ is the distance between the test mass $m$ and the infinitesimal element at $Q$.
Due to symmetry, straight lines through the center of the ring must be all equivalent.
For simplicity, select the line through the x-axis for measuring forces. Polar coordinates
are to be employed. Furthermore, let $\overline{QP}$ the perpendicular from $Q$ onto $\overline{mM}$.
Then, according to Pythagoras' theorem and some simple trigonometry:
$$
r^2 = (\overline{Mm}-\overline{MP})^2+(\overline{PQ})^2 =
\left[d-R\cos(\phi)\right]^2+\left[R\sin(\phi)\right]^2 \quad \Longrightarrow \\
r^2 = d^2-2Rd\cos(\phi)+R^2
$$
Due to symmetry, we only need to consider the component of the force that is directed from
$m$ to $M$, being a projection:
$$
\overline{Pm}/\overline{Qm} = \frac{d-R\cos(\phi)}{r}
$$
Herewith Newton's law becomes, with $r^3$ in the denominator:
$$
dF = G\frac{m\times\rho\,R\,dR\,d\phi}{r^2} \times \frac{d-R\cos(\phi)}{r} = m \times
G\frac{\rho\,R\,dR\,\left[d-R\cos(\phi)\right]\,d\phi}{\left[d^2-2Rd\cos(\phi)+R^2\right]^{3/2}}
$$
The total attractive force at the test mass, exerted by the ring, is the integral of this.
Due to symmetry, half of the computational effort can be saved.
$$
F = m \times 2 \int_0^\pi
G\frac{\rho\,R\,dR\,\left[d-R\cos(\phi)\right]\,d\phi}{\left[d^2-2Rd\cos(\phi)+R^2\right]^{3/2}}
$$
Make the result dimensionless as much as possible:
$$
F(d/R) = m \times G \rho\,dR/R \times 2 \int_0^\pi
\frac{\left[(d/R)-\cos(\phi)\right]\,d\phi}{\left[(d/R)^2-2(d/R)\cos(\phi)+1\right]^{3/2}}
$$
This formula is valid even for test masses inside the ring: not only for $d > R$
but also for $d < R$. The function $F(d/R)$ is depicted in the figure below. Function value
positive means that the force vector is directed from the left to the right. Function value
negative means that the force vector is directed from the right to the left.
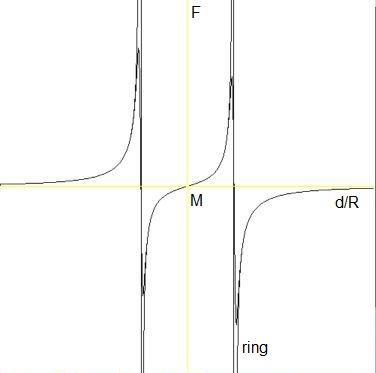
A special case is a test mass at the ring. Then the integral becomes, for $d/R=1$:
$$
F = m \times G \rho\,dR/R \times 2 \int_0^\pi
\frac{\left[1-\cos(\phi)\right]\,d\phi}{\left[1-2\cos(\phi)+1\right]^{3/2}} =
m \times G \rho\,dR/R \times \frac{1}{\sqrt{2}} \int_0^\pi \frac{d\phi}{\sqrt{1-\cos(\phi)}}
$$
Quite unfortunately, this special integral is singular; it has an infinite outcome,
because the denominator of the integrand is zero for $\phi=0$. How do we deal with that?
The answer is that we don't! Take a look at the above graph. If the test mass $m$ is close
to the ring, then it will experience a strong force towards the ring. This simply means that
a test mass cannot escape from its RingWorld. And that's all. A test mass in the ring does
not contribute to anything else than just staying there. Therefore what we shall do is:
simply skip / ignore the infinite contribution.
# Gravity constant (G)
G := 6.67408*10^(-11);
# Speed of light
light := 299792458;
# Number of seconds in a year
year := 31556926;
# Radius of milky way (L)
radius := 52850*light*year;
# Mass of our sun
sun := 1.989*10^(30);
# Mass of milky way (W)
mass := 1.5*10^(12)*sun;
# Speed of sun (V)
speed := 250000;
# Dimensionless number
G*mass/(radius*speed^2);
6.372018778
At this stage in the project, it is not quite important though, because we are only interested in
velocities relative to each other.
Crucial for success of the calculations is the (observed) mass density profile of the stars
in the galaxy. For a novice in the field, it's not easy to find something sensible
in the references available on the internet. After some search I've decided to take
this one, on page 19. In the figure below, the graph of this function is sketched in
$\color{red}{red}$. There is another scaling factor involved that norms the mass of the galaxy
to unity, according to $\int_0^1 \rho(R)\cdot2\pi R\,dR = 1$. Apart from such minor details,
this is what we finally have in the program:
$$
\rho(R) \sim \left[1+(R/\sigma)^2\right]^{-3/2} \quad \mbox{where} \quad 0 \le R \le 1 \quad
\mbox{and} \quad \sigma \approx 0.1
$$
I think that the conclusion is clear. If the adopted mass density profile of the stars is indeed
acceptable, then the galaxy rotation speed profile $v(r)$ is not according to the observations.
This means that I've not yet ( 2016 ) been able to reproduce the claims in the papers by
Kenneth F Nicholson and
James Q. Feng and C. F. Gallo.
Note. These papers
are deviant from the webpage you are reading in that they handle an inverse problem:
how to determine the mass density distribution from a given velocity profile. But still then there is no reason to believe
in a Deficient Reasoning for Dark Matter in Galaxies,
as far as I can see.
|
Further confirmation for this stand is found at The 100 Year Wrong Turn in Cosmology. Search for "(2010)" and find a (broken) link to the following publication:
Galactic Rotation Described by a Thin-Disk Gravitational Model without Dark Matter.
At least, that's what the title says. In the body of this PDF we are reading something else, though: However our predicted mass densities show generally slower decaying rates toward the galactic periphery than that of measured brightness, consistent with more ordinary baryonic mass in the outer disk regions having relatively lower light emissivity (and thus appearing darker). With other words: NOT without resorting to Dark Matter. Even more evidence is supplied by L. Marmet and the same C.F. Gallo: Comment on the use of Kepler's laws to describe galactic rotation. Quote: The excellent data on rotation curves of galaxies and high mass-to-luminosity ratios provide the main arguments in favour of "matter that has no light". And in Rotation Dynamics of a Galaxy with a Double Mass Distribution, by Louis Marmet alone, we read: It is proposed that dark matter does not have to be exotic non-baryonic matter. Instead, it can be made of molecular hydrogen and condensed matter. Can't resist: it must be condensed milk then, because we are talking about a milky way, right? Look! The James Webb Space Telescope shows that there is spilled milk all over the place (-: |  |
Whatever. It all simply means: WITH dark matter. It seems to me that there hasn't been accomplished much here.