Evidence once more

$ \def \EN {\quad \mbox{and} \quad} \def \hieruit {\quad \Longrightarrow \quad} $ In a previous section, called 'Quotient Function', evidence has been gathered for the following theorem: $$ \frac{b}{a}(dx) = e^{\, \left[ b/a \right]'(0) \: dx} $$ I feel not entirely comfortable with the "proof" in this section, though. The task could be re-formulated as follows: given $f(2.x) = f(x).f(x)$, continuous in $x$, prove the theorem that: $f(x) = \exp(Px)$. It is clear that the theorem is a sufficient condition for $f(2.x) = f(x).f(x)$ being true. But is it also a necessary condition? Up to now, the proof has been accomplished by - sort of - transfinite induction. Truth has been established for grid spacings approaching zero - the domain of Calculus - and then, while working backwards, for finite sized grid spacings, like in Numerical Analysis. In terms of Chaos Theory: the theorem is proved for the Analytical Attractor in a/b(0) = 1 and then very much extrapolated. Therefore trying to arrive at the same result via some other road seems to be worthwile the effort.
The end-result from the section 'Direct Solver' is recalled in the first place: $$ \left[ \begin{array}{ccccccc} . & . & . & & & & \\ & & . & . & . & & \\ & \displaystyle - \frac{a_{54}}{a_{55}} \frac{a_{43}}{a_{44}} & 0 & \displaystyle 1 - \frac{a_{54}}{a_{55}} \frac{a_{45}}{a_{44}} - \frac{a_{56}}{a_{55}} \frac{a_{65}}{a_{66}} & 0 & \displaystyle - \frac{a_{56}}{a_{55}} \frac{a_{67}}{a_{66}} & \\ & & & . & . & . & \\ & & & & . & . & . \end{array} \right] \left[ \begin{array}{c} . \\ . \\ T_3 \\ T_4 \\ T_5 \\ T_6 \\ T_7 \\ . \end{array} \right] $$ Thus we see that the off-diagonal coefficients of the coarsened grid are given by: $$ a'_{53} = - \frac{a_{54}}{a_{55}} \frac{a_{43}}{a_{44}} \EN a'_{57} = - \frac{a_{56}}{a_{55}} \frac{a_{67}}{a_{66}} $$ Independent of the kind of mesh involved. Now imagine a piece of an 1-D mesh again, but this time with a non-uniform grid spacing:

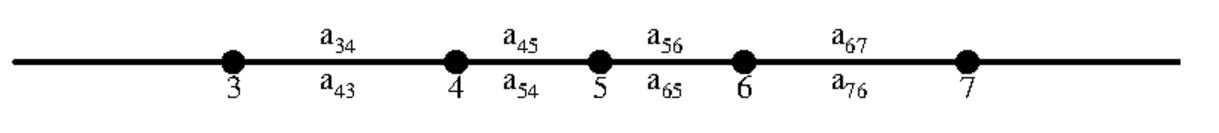
Where we have arranged it in such a way that the distance between the vertices $(3)$ and $(4)$ is equal to the distance between the vertices $(6)$ and $(7)$; and the distance between the vertices $(4)$ and $(5)$ is equal to the distance between the vertices $(5)$ and $(6)$. Furthermore it is assumed that the matrix coefficients are only dependent upon these two distances, called: \begin{eqnarray*} \mbox{distance }_{3-4} = \mbox{distance }_{6-7} = dx_1 \\ \mbox{distance }_{4-5} = \mbox{distance }_{5-6} = dx_2 \end{eqnarray*} If matrix elements which are at the left of the main diagonal are denoted as $a$ and matrix elements which are at the right of the main diagonal as $b$, as has been done before, then it becomes readily evident that: \begin{eqnarray*} a_{43} = a(dx_1) \EN a_{54} = a(dx_2) \\ a_{67} = b(dx_1) \EN a_{56} = b(dx_2) \end{eqnarray*} Furthermore it follows that $a_{44} = a_{66}$ and: $$ a'_{53} = a(dx_1 + dx_2) \EN a'_{57} = b(dx_1 + dx_2) $$ Repeat: $$ a'_{57} = - \frac{a_{56}}{a_{55}} \frac{a_{67}}{a_{66}} \EN a'_{53} = - \frac{a_{54}}{a_{55}} \frac{a_{43}}{a_{44}} $$ Hence the quotient of the off-diagonal coefficients of the coarsened grid can also be written as follows: $$ \frac{b(dx_1 + dx_2)}{a(dx_1 + dx_2)} = \frac{b(dx_1)}{a(dx_1)} \frac{b(dx_2)}{a(dx_2)} $$ Herewith it is established that the quotient function is characterized by: $$ \frac{b}{a}(dx_1 + dx_2) = \frac{b}{a}(dx_1) \frac{b}{a}(dx_2) $$ And we are triggered to pay some attention to functions which are characterized, in general, by the following property: $$ f(p+q) = f(p) . f(q) $$ A couple of other properties can be derived herefrom: $$ f(q) = f(q-p+p) = f(q-p) . f(p) \hieruit f(q-p) = f(q) / f(p) $$ $$ \hieruit f(p-p) = f(p)/f(p) \hieruit f(0) = 1 $$ Employing the hypothesis that $f$ is a differentiable function and taking the limit for $dx \rightarrow 0$, we see that: $$ f'(x) = \frac{f(x+dx) - f(x)}{dx} = \frac{f(x).f(dx) - f(dx)}{dx} = $$ $$ f(x) \frac{f(dx) - 1}{dx} = f(x) \frac{f(dx) - f(0)}{dx} = f'(0).f(x) $$ Giving a differential equation for $f(x)$ which can be solved rather easily: $$ \frac{df}{f} = f'(0).dx \hieruit ln(f) = f'(0).x + c \hieruit f(x) = C e^{f'(0).x} = e^{f'(0).x} $$ Where the boundary condition $f(0) = 1$ is employed in the last step. $f'(0) = P$ is an arbitrary constant. The result is in agreement with our conjecture that the only possible Quotient Functions are, indeed, given by: $a/b(dx) = \exp(P.dx)$. If the above proof is valid, then this conjecture has been turned into a theorem.