Uniform Probability

Questions & Answers @ Mathematics Stack Exchange:

From that internet page about Natural Densities :
Quote: We see that this notion can be understood as a kind of probability of choosing a number, which obviously is the reason why Natural Densities are studied in probabilistic number theory. No big surprise therefore that another application of the above concepts is with Probability Theory.
Standard mathematics says that it is impossible to have a uniform probability distribution on the naturals. Meaning that it is impossible to have a distribution giving equal probabilities to each of (all) the natural numbers. Not a question, however, about the same for an initial segment of the naturals. The probability for picking, say, the number $7$ out of the initial segment $\{1,2,3,4,5,\dots,n\}$ is simply: $1/n$. The same holds for an arbitrary number $k$ in that segment. And the sum of all the probabilities is $1/n + 1/n + \dots + 1/n = n.1/n = 1$, as it should. But something weird happens if we take the limit for $n \rightarrow \infty$. Then each of the probabilities for picking one natural number becomes $$\lim_{n \rightarrow \infty} 1/n = 0$$ while the sum of all probabilities is still $1$, according to $$\lim_{n \rightarrow \infty} 1 = 1$$ How can this happen? A bunch of zeroes that sums up to one? Oh well, it's not just a sum of zeroes. It's an infinite sum of zeroes. But nevertheless.
Let's take another example, that only seems remotely resemblant. The following very simple integral is expressed as the limit of a Riemann sum: $$ \int_0^1 dx = \lim_{n \rightarrow \infty} \sum_{n=1}^n 1/n = \lim_{n \rightarrow \infty} n.1/n = \lim_{n \rightarrow \infty} 1 = 1 $$ Am I blind or what? The only difference with the above "probabilities" is the geometrical interpretation. Here come the probabilities. It is a histogram with height of the blocks $1/n$ and width of the blocks $1$ for $n$ blocks. So the total area of the blocks is $(n.1.1/n) = 1$ :

And here comes the Riemann sum of the trivial integral. The blocks are $1/n$ wide, $1$ high and there are $n$ of them. The total area is $(n.1/n.1) = 1$.

Quite obviously, $\;\lim_{n \rightarrow \infty} n.1/n\;$ is exactly the same algebraic expression with the integral as with our probabilities. But, on the the contrary, it seems that common mathematics has developed quite different ideas about probabilities. The following is a standard Theorem.

We can prove there is no uniform distribution on $Q$ .

Proof. Assume there exists such a uniform distribution, that is, there exists $a \ge 0$ such that $P(X = q) = a$ for every $q \in Q$ .
Observe that, since $Q$ is countable, by countable additivity of $P$ : $$ 1 = P(X \in Q)=\sum_{q \in Q} P(X = q) = \sum_{q \in Q} a $$ Observe that if $a = 0$ , $\sum_{q \in Q} a = 0$ . Similarly, if $a > 0$ , $\sum_{q \in Q} a = \infty$ . Contradiction.
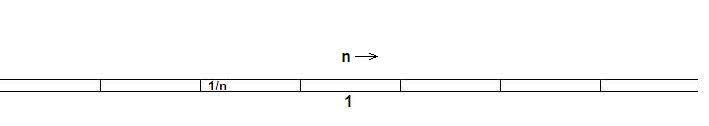
Let's analyze. The second part of this reasoning is remotely resemblant to the following iterated limit: $$ \lim_{n\rightarrow\infty} n \left[ \lim_{m\rightarrow\infty} \frac{1}{m} \right] = \lim_{n\rightarrow\infty} n\; 0 = 0 $$ First define uniform probabilities. It's easy to see that these will become zero if the initial segment becomes infinite. Then sum up. Evidently the sum then must be zero as well. We have learnt, though, that iterated limits may be understood in a quite different way. Due to the following Theorem, proved elsewhere by this author: $$ \lim_{x\rightarrow a} \left[ \lim_{y\rightarrow b} F(x,y) \right] = \lim_{(x,y)\rightarrow (a,b)} F(x,y) $$ Translated to the present case of interest, with $F(m,n) = n.1/m$ : $$ \lim_{n\rightarrow\infty} \left[ \lim_{m\rightarrow\infty} n.\frac{1}{m} \right] = \lim_{(m,n)\rightarrow\infty} \left[ n . \frac{1}{m} \right] $$ However, the numbers $m$ and $n$ must be equal, $m = n$ , because they denote one and the same upper bound for the initial segment of the naturals at hand. Therefore we actually have: $$ \lim_{n\rightarrow\infty} \left[ n . \frac{1}{n} \right] = \lim_{n\rightarrow\infty} \left[ 1 \right] = 1 $$ I think the twist is clear now. But ah, the trouble with understanding all this might be something else as well ..
The sum of infinitely many zeroes in our tentative probability theory is equal to one. The Riemann sum in infinitesimal calculus - mind infinitesimal - is equal to one as well. Could it be that the existence of infinitesimals is consequently denied in common mathematics - because, especially in calculus, they "aren't needed anymore". But as soon as we add a twist to probability theory, it seems that they turn up again.
As has been said in the beginning, our tentative probability theory is actually equivalent with Natural Densities. The probability that a natural number is e.g. seven is exactly zero, just meaning that the density of seven in the naturals is zero. Yet the density of a natural being an even number is not zero: it equals $1/2$. In fact, it has no sense to distinguish Uniform Probabilities on the Naturals from Natural Densities from Finitary Cardinalities. Maybe somewhat exaggerated, but one gets the idea. The redundancy, as a consequence of Infinitum Actu Non Datur may be summarized loud and clear: $$ \mbox{Finitary Cardinalities} = \mbox{Uniform Probabilities} = \mbox{Natural Densities} $$